 |




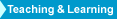















|
 |
 |
|
author:
|
Andee Rubin
|
|
description:
|
This paper considers a wide variety of educational software resources and their educational effects in considering the questions: How can educational technology support children's learning? Are there different uses of educational technology that fit with various theories and types of learning? What are the emerging trends in educational technology that respond to new understandings of how students learn?
|
|
published in:
|
paper from "Technology Infusion and School Change," TERC
|
|
published:
|
05/01/1996
|
|
posted to site:
|
06/11/1998
|
INTRODUCTION
HOW CAN EDUCATIONAL TECHNOLOGY SUPPORT STUDENTS' LEARNING? Are there different
uses of educational technology that fit with various theories and types of
learning? What are the emerging trends in educational technology that respond to
new understandings of how students learn? This paper investigates one approach to
answering these questions, by considering a wide variety of educational software
resources and their educational effects.
The number and variety of educational software titles available to schools and
homes has increased dramatically over the last several years. Just the titles and
sources of current programs fill an entire CD-ROM, The Educational Software
Selector (TESS) (Epie Institute, 1991). To discuss educational software in its
full scope, some organizational scheme is necessary. TESS uses an approach that
sorts by academic content, grade level, and classroom organization. The
categories used herein relate to the uses of educational software to support
student learning.
Until recently, much of educational technology has been used to support the
teaching and learning of basic skills such as arithmetic facts, spelling, and
other topics that could be reduced to multiple-choice questions. However, recent
research on cognitive growth and learning, building on the views of Dewey,
Piaget, Vygotsky, and others, has led many educators to reconsider teaching
approaches in general, and the ways technology is used to support student
learning in particular. These new perspectives on student learning form the
pedagogical basis of recent and newly emerging standards for mathematics and
science teaching and learning. Although these standards documents differ in
detail, they all have at their core a common view of the value of a particular
kind of learning, which I call "inquiry-based learning." This paper focuses on
inquiry-based learning for two reasons. First, because our current understandings
of education highlight its importance, specifically as the most powerful mode in
which students learn. Second, as our understanding of learning has evolved, so
has our understanding of how technology can support learning; it has become
apparent that technology is particularly well-suited to support inquiry
learning.
This paper contains two major sections. The first section describes inquiry-based
learning in some detail and technology's potential role in supporting it. This
section also includes several general points about the ways in which technology
and learning interact in schools, as well as inherent difficulties in assessing
the results of introducing a combination of technology and inquiry into
classrooms. The second section then presents categories of software organized
around their relationship to inquiry-based learning. Besides identifying groups
of software that have similar underlying positions with respect to inquiry, this
section includes several specific examples that illustrate the kinds of thinking
and problem solving that students might engage in while using this software.
INQUIRY-BASED LEARNING, WITH AND WITHOUT TECHNOLOGY
This section defines inquiry-based learning and describes key elements and
characteristics, whether or not technology is used. It goes on to suggest ways in
which technology can support inquiry-based learning, and closes with suggestions
for evaluating the educational effects of this approach to teaching and
learning.
Defining Inquiry-Based Learning
What is inquiry-based learning? There are as many specific answers as there are
people to ask, but there are common themes to the descriptions that represent a
core of belief about inquiry. The list below is loosely based on Nickerson
(1988), but includes some additional thematic elements and some connections to
technology.
Constructivism. The major claim of this theme is that learning is an active
process, described as forming new mental models rather than as assimilating
information. Students continually create their own mental models as they
encounter new material. It is questionable if "passive learning" could even
exist. Integral to the concept of constructivism is the notion that much of
learning comes from grappling with complex problems, for which there may be
multiple approaches. The interaction a learner has with others engaged in the
task adds to the learning potential; language is the most important carrier of
these inquiry-supporting interactions. Out of such experiences, learners build
their own knowledge.
Importance of conceptual understanding, rather than procedural efficiency.
Especially in math and science, much of the knowledge students are often expected
to know is procedural; that is, how to follow particular rote recipes. If this
knowledge is not situated in an understanding of how and why the procedures work,
students may not be able to know when and how to use them. Conceptual
understanding includes a much richer and more flexible array of knowledge that
makes it possible for students to think deeply even without a procedure, to know
when and how to apply proper procedures, and to interpret their results
appropriately.
Responsiveness to what students already know. No student enters a class as an
empty vessel. Education must take account of what students bring with them. Based
on life and school experiences, every student has formed many ideas about math,
science, social studies, writing, etc. Some of these pre-existing ideas are
valuable bases for continued learning; others are wrong and would lead the
student further into territory that is not educationally useful. Students'
incorrect ideas have sometimes been called "misconceptions" and inquiry-oriented
methods to help students reform their ideas into more correct conceptions have
been designed. Technology can play a role in this regard by assisting teachers in
understanding students' knowledge and current conceptions, as many pieces of
software help students display their thinking and procedures in a more accessible
form.
Connections to the world outside of schools. Research is beginning to show that
one problem with school learning is that students often fail to connect it to
what they have learned outside school. Students often bring knowledge to class
that is directly relevant to what they are learning, but fail to see the
connection. In response to this issue, some of the new curriculum efforts are
focusing on the creation of authentic tasks which meet needs and goals that
students either have already or might have in the future.
Furthermore, students often fail to see how the work they do in school is related
to their lives at home. Parents can do much to support home-school connections,
but research has documented most parents' lack of connection with their
children's schools. Chris Dede (O'Neil, 1995) claims that "We know that the
biggest single impact that we could make in the lives of many children would be
to involve their parents more deeply in their learning" (p. 10).
Metacognition. Students need to know how to take responsibility for managing and
monitoring their own thinking and learning activities. These kinds of skills
(e.g., knowing when you have learned something or planning to use your most
effective learning strategies to master some content) are sometimes called
"metacognitive skills" because they require the students to examine their own
learning practices. In an inquiry-based perspective, students need to reflect on
the steps they take to generate questions about a new topic, how they collect
information to help focus on a smaller set of questions, how they evaluate the
relevance of the information, how they decide to what steps to take next, and how
they communicate their conclusions. Unfortunately, most curricula do not
explicitly call for a focus on metacognitive learning.
Lifelong learning. The students of today will need to learn throughout their
lives. In the past, technology and jobs changed relatively slowly, but today's
world can change practically overnight. Many of today's jobs require facility
with technologies that didn't exist 20 years ago, and reeducation is the only way
some people can continue to work at skilled jobs. Students need to prepare in
school to continue to learn for the rest of their lives; in terms of inquiry,
this means cultivating curiosity, knowing where learning resources might be,
having experience with tacking complex problems, and knowing how to work with
others in crafting approaches to difficult situations.
What does a classroom in which inquiry is taking place look like? Commonly, some
or all of the following characteristics are present:
- Questions are, in general, complex.
- Answers to questions are open-ended.
- Most questions have more than one right answer or more than one way to get to a right answer or both.
- Students are assessed by how they get to the answer, as well as the answer itself.
- Discussion among students or between students and teacher is part of the process.
- Students have to plan and organize as part of their work on a problem.
- Communication takes place in multiple modalities and forms--both oral and written, pictorial, graphical, and symbolic.
- Teachers play a role as facilitators of learning, rather than as transmitters of information.
Technology in Support of Inquiry-Based Learning
The descriptions above mention nothing about technology for, in fact, solid
inquiry-based learning can occur without technology and often does. But
technology can play a special role in supporting inquiry-based learning and in
transforming classroom practice. To better understand the context in which
technology can support inquiry-based learning, two important distinctions should
be noted: technology can be viewed as the subject of instruction or as a tool for
instruction, and can serve as an amplifier of traditional practice or as a
transforming agent. These varying approaches are discussed below.
Learning with computers, not learning about computers. We distinguish between
learning about computers (e.g., how to hook up a disk drive, what is the
difference between RAM and ROM) and learning with computers. This paper focuses
on learning with computers; that is, learning the topics of the curriculum
(language arts, math, science, social studies, etc.) with computers as a
pedagogical tool. This does not deny that students need to know how to attach
peripherals, put in and use a digitizing card, be proficient in a programming
language and so on. But except for a general introduction to the way the machine
operates, students tend to pick up most of these skills naturally in the context
of using computers to learn other subjects. To learn effectively with computers,
a few skills (e.g., keyboarding) are necessary in advance. Some students may need
to spend time focusing on these skills to avoid frustration. But even keyboarding
is best learned in the context of using the computer for other tasks, so that it
does not become a rote lesson without a purpose.
The answer to the central question, "Will technology have a significant effect on
K-12 education?" can be determined only by asking a follow-up question: "How is
the technology used by students and teachers?" Their experiences, in turn, are
determined by the models of teaching and learning that underlie the instruction
in their classrooms. Pedagogy is the key. When we look at the interaction between
pedagogy and technology, the most obvious conclusion is: Traditional pedagogy
isn't improved much by the addition of technology. Good pedagogy, on the other
hand, can be made significantly more effective by appropriate uses of
technology.
When learning with technology focuses on doing inquiry-based learning, the
following approaches are commonly adopted in classrooms:
- Technology is viewed as a tool, much like a pencil or pen, but considerably more powerful.
- Use of the technology is primarily taught in the context of solving problems.
- Students help one another with the mechanics of the technology; in fact, in many classrooms, students are the local experts on technological details.
- Talk about and around technology is as important as the technology itself, just as talk about how one finds and uses information is as important as the information itself.
- Technology is used to augment communication by expanding audience (e.g., over networks and by producing hard copy) and expressive options (e.g., mixing graphs and words).
Amplifiers and transformers. It is helpful to distinguish between two broad
categories of use of educational technology: amplifiers and transformers.
Technology is used as an amplifier when it makes a current practice more
efficient or effective. The same technology can be used as a transformer if it
changes the way a topic is examined, the social structure in the classroom, or
the process by which students carry out a complex task. Word processors are a
good example of a technology that can be - and often has been - used in both
ways. A word processor is used as an amplifier when students write drafts on
paper, copy them onto the word processor and edit spelling, and grammar, for
instance, on the word processor. Because of resource limitations, this is often
the way word processors are used in schools. There are positive educational
effects to this use - students can correct their work without rewriting or
retyping, they can see their errors more easily in type than in handwriting,
their final product is easier for others to read - but it does not change the
writing process in significant ways.
A more transformative way to use word processors would resemble ways in which
adults use them - to take notes, to fashion individual phrases and sentences, to
construct a final draft, and to revise by moving words, phrases, sentences, and
paragraphs around. This learning process might be embedded in a social setting in
which students comment on one another's papers (perhaps for publication) and
revisions are made in response to others' suggestions. In this scenario,
students' writing processes are changed in a significant way from a more
traditional approach. The introduction of a real audience and purpose - as in
publishing a class literary magazine - has transformative potential, as the
writing process changes in response to the authenticity of purpose and audience.
Word-processing technology makes this audience or purpose more accessible, but
does not in and of itself provide or mandate it. That is the job of the teacher
and the curriculum approach.
A final comment about the amplifier/transformer distinction: In classrooms that
have already been "transformed" by curriculum innovations (e.g., the Writing
Project), what might in other classrooms be considered a transformative use of
technology might here be considered an amplification of the current curriculum.
Therefore, in a classroom that has a serious commitment to the writing process,
adding word processors would serve to amplify - in quite significant ways - the
current classroom practice. The technology will do less to transform the
curriculum approach in this setting than it would it a classroom still working
with more traditional writing instruction. In applying the terms amplifier and
transformer, therefore, we must always be clear about what is being amplified or
what is being transformed. The use of each of these terms is relative to the
choice of a starting point from which to proceed.
Understanding How Technology Has Affected Student Learning
Given that technology's effects depends on its uses, rather than simply on
characteristics of the software, how do we assess and evaluate its effect?
Teachers, principals, parents, and policymakers care deeply about the answers to
this question. Such evaluations are often used to decide whether a technology
program has been a success, to compare schools systems that have embarked on
different technological paths, or to decide whether to continue to support a
technological specialist, in addition to the traditional uses of assessment to
compare students, teachers, and schools. At this general level of discussion,
there are three important principles that reinforce the complexity of the task of
measuring educational effect. Consider the following:
Educational change takes time and is a complex process. Although some educational
change can be relatively rapid, inquiry-based reforms are especially likely to
take significant amounts of time. Inquiry innovations that use technology require
sufficient time for teachers to master the tools and the pedagogy to the extent
that they can structure the classroom so that inquiry emerges. Research supports
these insights. Most of the studies have found that changes in student learning
as a result of some innovation take several years to become measurable. Some
research reports that, with daily use of technology, it is at least a year before
any changes are evident. Where educational goals are focused, relatively
contained, and connected closely to assessment methods, changes can be seen in
periods of one to two semesters (Herman, 1994). But where more significant
restructuring is taking place, periods of several years are more realistic
estimates for seeing significant changes in student learning.
Where major changes in pedagogy are involved, one needs to take into account the
amount of time professional development requires, both for actual
out-of-classroom activities such as workshops, study groups and in terms of the
rate at which personal change (for that is what pedagogical change entails) takes
place (see Grant, p. 72). The important point is that measuring student learning
variables too early may suggest that little is happening - when in fact it is
just too early in the process to see results.
Choices of assessment method - test scores, projects, portfolios - and content -
facts, methods, inquiry - will determine what "changes" are observed and valued.
Measuring changes in student learning based on technology use is a serious
challenge. Besides the necessity of allowing sufficient time for change, there is
the question of choosing an appropriate measure. In the past, often the only
measure available was standardized tests. They are convenient because they are
administered routinely, norms exist, and scores are reportable for students,
classrooms, schools, districts, etc. These tests are predominantly
multiple-choice (and thus easily scorable) and tend to focus on small, definable
subskills and fact recall.
In the last decade or so, in parallel with the development of new standards,
particularly for math and science learning, radically different approaches are
being taken to assessment. Some of the work on both standards and assessment in
math, for example, was in response to the observation that students in our
educational system perform adequately on simple computational problems but
stumble on problems that require them to figure out how to organize the
operations rather than just carry out a designated set of steps. In general, the
kind of test questions on which students do worst are those that require an
understanding of the context of the question, not those that are straightforward
arithmetic. A typical problem might be the following: "How many 60-pound dogs
does it take to balance a 3,000-pound elephant?" (Mokros et al., 1994). Many
students, lacking an understanding of the mathematics of the problem, try all
four basic arithmetic operations using 3,000 and 60, but have no way of
evaluating which of the four answers is correct!
Critics have pointed out that standardized tests reflect an earlier view of
learning in which quick performance of short, repetitive tasks was the most
important skill to measure. As the demands of the job market, our knowledge of
the variety of people's intelligences (Gardner, 1983) and our view of schools'
purposes change, these kinds of tests have become less relevant. Organizations
such as the New Standards project, National Council of Teachers of Mathematics,
and National Assessment of Educational Progress are all working on new styles of
assessment that reflect changing educational values. Such tests, in general, ask
more open- ended questions that require students to plan and organize a
problem-solving approach, rather than answer a large number of multiple-choice
questions. Scoring these tests, obviously, is more complex than machine-scoring
of the older style of tests.
In general, the more closely an educational innovation (technological or not) is
linked to the measures used to evaluate it, the more likely it is to show
measurable results. Making this link is the easiest with very specific,
out-of-context skills like "knowing the multiplication tables." But conceptual
change, such as that implied by inquiry-based learning, is more slippery. Because
the education we desire seeks the acquisition of a wide variety of capabilities:
"domain-specific knowledge, generally useful cognitive skills, and the ability
and desire to learn," (Nickerson, 1988, p. 3), the results are harder to measure.
Thus, for inquiry-based learning in general, and that with technology in
particular, statistical measures of effectiveness are often lacking. Furthermore,
this is compounded by not having enough funding to carry out multiyear studies.
Herman (1994) has suggested methods for more effective assessment strategies that
include merging quantitative and qualitative methodologies, building and
assessing theories of action, using process indicators as proxies for student
outcomes, etc. This is the kind of work that will need to be expanded if we are
to document how technology supports student inquiry.
What counts is what happens in the classroom, not what is written in the manual.
And these may be quite different. In looking at the educational effects of
technology, we must distinguish between the intention of an innovation - what its
developers envisioned - and what really happens in a classroom. It should be no
surprise that what actually happens with the same technology and the same
curriculum differs from classroom to classroom. To be realistic about technology,
we must keep in mind the variety of experiences that may take place with
different teachers.
There is a complex relationship between the design of a technological innovation
and what happens in a classroom. The developers have in mind some "idealization"
of a technological innovation, embodied in its software and accompanying
instructions for its use, which often include a large number of pedagogical
assumptions. In using the software, however, each teacher creates a different
realization, specific to his or her beliefs and implementation process. It is
important to keep this "realization" and adaptation process in mind in evaluating
any technological innovation; one central point of comparison is between the idea
the developers tried to communicate and the innovation the teacher implemented
(Bruce & Rubin, 1993). As one paper about the use of some computer-based writing
tools in classrooms states, "The computer is the dependent variable." As such, it
is subject to the pedagogy and beliefs of the teacher and to the specific
circumstances of the classroom (Michaels, 1990).
These differences are particularly important in thinking about open-ended
software that intends to support open-ended, student-centered activity; such
software, by its very nature, cannot control what happens in the classroom. As
always, it is the teacher who controls the way it is used. Thus, teachers who
want to have classrooms in which all students are working on learning the same,
identifiable skills, can just as easily use open-ended software as part of that
traditional structure. One of the apocryphal (but true) stories told in the
educational technology community is how many teachers developed worksheets to
accompany LOGO®, software whose underlying philosophy and structure was dedicated
to students' designing their own projects. These teachers in a sense turned the
use of LOGO into the study of LOGO - and saw their educational goals as the
answers to questions such as, "Write the command to make the turtle go forward
and back 10 steps." This tendency to modify the intention of open-ended software
is widespread in many schools, where there is tension between the old and the new
ways of teaching, with pedagogical change occurring at the same time that
technology is being introduced.
CATEGORIES OF SOFTWARE USE
Beyond these general descriptions, there is much more specific information about
how technology can support inquiry in the classroom. Because different pieces of
software support inquiry in different ways, the technology descriptions below are
grouped into twelve categories, primarily by how they affect classroom practice.
The discussion of each category includes a determination of how it does - or does
not - support inquiry, a look at how the software might be used in support of
inquiry-based learning, what classroom interactions might look like, and how and
what students might be learning. In some cases, a more specific vignette is
included to illustrate how the software-student interaction might proceed.
A general comment on these categories: It is difficult to categorize anything
complex into a small number of mutually exclusive categories. Many of the pieces
of software described below belong in the intersection of several categories; in
fact, the best software combines characteristics from many categories and can
greatly increase its power and effect in this way. In some ways, therefore, these
categories describe characteristics of software that can be combined in many
ways. Furthermore, educational uses of telecommunications technologies bring
additional opportunities to extend computer usage in creative ways that support
inquiry- based learning. These applications are not described in detail here, but
can incorporate many features noted in this categorization. (For further
discussion of a range of uses of telecommunications networks, see Berenfeld,
1996).
The categories are:
- Generic information handling tools
- Real-time data acquisition/MBL
- Simulations
- Multimedia
- Educational games
- Cognitive tools
- Intelligent tutors
- Construction environments
- Virtual communities
- Information access environments
- Information construction environments
- Computer-aided instruction and integrated learning systems
In particular, two characteristics of software use are orthogonal to the
categories above, but they are at least as important in their effect on student
learning. First, the potential for software to change the social context in which
it is used is significant; for example, to create the opportunity and the content
for discussion among students or between teachers and students. Much of the
student learning with these tools arises primarily from student collaboration in
joint problem solving. The conversation that takes place between students in
their construction of a joint understanding of a problem can be both a catalyst
and an opportunity for them to clarify and add to their knowledge. Tom Snyder's
Search Series, for example, works with students in teams that might make
collective navigation and resource decisions and discover minerals or oil spills.
Much of the learning takes place as students defend their ideas for the next
move.
Second, many of the pieces of software described below can be used in support of
students' work on authentic, complex tasks. Tasks that involve research,
communicating with experts, recording and representing information, presenting
results, and persuading audiences reflect the kind of work students will engage
in throughout their careers. These tasks are in general multidisciplinary and
require collaboration, as are most tasks in real life. One such task might be to
design a garden for the schoolyard and present the results to the rest of the
school; this project would involve art, language arts, math, science, and,
perhaps, even social science. Technology to support it could range from geometric
analysis programs to drawing programs to the World Wide Web for finding
information on plants and seeds to word processors for preparing the report.
Finally, this paper includes a special section on computer use in multicultural
education because the topic of equity is of major importance. The categories of
useful software are no different in multicultural classrooms, but certain uses
have appealed to teachers as especially effective in allowing students to master
complex technology and to feel they have found their "voices" through the special
qualities of certain pieces of educational software.
1. Generic Information-Handling Tools
Almost everyone who has ever used a computer has used one of the basic
information-handling tools: word processors, spreadsheets, databases, graphics
packages, and page layout packages. Most adults who use computers in their jobs
or at home use some or all of these tools. All these tools provide enormous
flexibility for manipulating information once it is entered into the computer.
Word processors can support flexible writing, in which multiple drafts are easy
to construct, spelling and word choice can be supported by computer tools, and
printed products have an "official" look that commands more serious reading by
parents and friends. For some students, just the move away from handwriting to a
more readable text is a significant boost in writing practice.
Data-handling software - spreadsheets and data bases - are similar in the
flexibility they support. Students can see several different graphs of a data set
in just a few minutes, expanding the ways they can conceptualize and understand
their data. They can look at derived functions of numerical sequences and graph
the results, allowing them to explore how the formula for a parabola is related
to its graph or how a sine curve is built. Sophisticated students can use
database and statistical functions to explore relationships among variables and
to determine what might be an important parameter of an identified effect. With
an appropriate curriculum, these tools can support inquiry as students explore
complex problems, take data, find meaning in their data, and write about their
findings. Most current word processors support the production of reports by
allowing students to integrate words, graphs, and spreadsheets into a single
document.
Many of these tools are used by real practitioners engaged in real
problem-solving situations. To be most effective, use of the tools in schools
should mirror as much as possible their use in real jobs. Examples of these
"adult" tools often used in educational settings are: Microsoft
Word®,Wordperfect®, Excel®, 1-2-3®, MacDraw®, PageMaker®, and FileMaker®.
The area of productivity tools has seen enormous growth over the last few years
as computers have become less expensive and more powerful, and as more and more
businesses provide workers with personal machines. This basic tool category could
arguably be said to include three-dimensional visualization software (e.g., CAD),
symbolic manipulation engines (e.g., Symbolica®), and sophisticated paint
programs (Studio 8®). For high school students in particular, any of the new
tools that are developed for the world of work may be relevant to learning in
some subject. In most cases of using these adult tools, however, it takes a
significant amount of time for students to learn the tool. If there are similar
tools designed with simpler interfaces and a more scaled-down set of functions,
it is often better to use them in schools. The trade-off to keep in mind here is
the power of the tool compared with the overhead involved in students learning
how to use it.
A second group of tools widely used in school are based conceptually on those
used in out-of- school applications, but are tailored for educational purposes.
To prepare software for an educational environment, the interface is simplified,
the most complicated functions may be eliminated and more helpful instructions
may be added. The Cruncher, for example, is a spreadsheet designed for elementary
school students, and the Wonderful Writing Machine is a word processor for young
students.
A third category of information-handling tools are those based on a completely
different design than adult tools, because their designers believe that a
different approach is more educationally appropriate. Tabletop, for example,
takes a unique approach to databases by representing each data point individually
rather than aggregating them, and by animating the process of constructing a
graph. Consider a database of countries with information on the population, GNP,
birth rate, average age, population, population density, etc. of each. In
Tabletop, each country would be represented by an icon - say a small flag. The
student can specify a scatterplot graph such as population by population density
using a very simple interface. The flags then march into their appropriate
positions along the two axes. The animation both captures students' attention and
shows graphically how points are positioned on a two-dimensional graph. When the
icons are all in place, the student can read the values of any of the other
variables (e.g., birth rate) in a simple way, such as by labeling each of the
flags with the value of this third variable.
Tabletop also includes some features that support inquiry in subtle but effective
ways. One of these is the ability to build up a slide show of interesting data
displays that students can use to show and discuss their work with other students
or teachers. This feature supports the conversations that are so important to
students becoming more practiced at inquiry. The slide show not only frees
students from remembering all the details of intermediate steps, but provides
illustrations for them to refer to in reflecting on and explaining their work
later.
In addition, there are sometimes parts of these tools that are "strictly
educational," software that demonstrates the meaning of a concept, but does not
directly contribute to the functionality of the system. Statistics Workshop
(Rosebery & Rubin, 1989), for example, is a statistical package that performs a
subset of the functions a full-fledged statistics package would offer. But it
also contains several explicitly educational parts. One, called "Shifty Lines,"
allows students to move a regression line on a scatterplot to see how different
positions and slopes offer better or worse fits to data. Another, called
"Stretchy Histograms," allows users to change a distribution and see how the mean
and median change as a result. The picture below illustrates how feedback on mean
and median of a distribution is given to the user as the distribution is
modified.
One or more of the tools described in this section can form the basis for a
curriculum innovation that enhances student learning. Even though each of the
tools on its own may have potential for educational change, it is the combination
of them in the context of a rich curriculum that produces real change. One
example of this process is the Immigrant 1850 project (Walters & Gardner, 1990).
Students had access to a core set of computer-based activities through which they
adopted an immigrant family and simulated the complex decisions the family had to
make in finding housing and a job. They used databases, spreadsheets, and word
processors to calculate their expenses, keep diaries, choose jobs, decide on
transportation, and see how their future turned out.
2. Real-Time Data Acquisition/Microcomputer-Based Labs
One of the most distinctive tools in the grab bag of educational software is
real-time data acquisition technology - also called generically
microcomputer-based labs (MBL). These various tools allow students to take data
in real time from action in the real world, and to record and analyze it. Not
only do students have the ability to investigate how the world works, but they
also learn how certain phenomena translate into graphical representations. An MBL
comes with a set of probes - each specified for a kind of measurement such as
distance, heat, temperature, heartbeat. Through a uniform interface, each of
these attaches to the computer, feeding information into spreadsheet and graphing
programs. The power of the MBL derives from its connection with events in the
students' world, with their motions, their heartbeats, their temperatures. The
simultaneous perception of the real-world event and its representation on the
computer can help students make sense of graphs and of the patterns they
depict.
In one of the more common applications of a MBL, students learn how graphs of
position, velocity, and acceleration are related and how they reflect different
motions in the real world. For example, students might run at different speeds
toward and away from a distance probe, creating a variety of graphs. They might
then figure out how to match each graph with one of their motions, predict what
velocity or acceleration graphs might look like or try to make a particular graph
by moving in the appropriate way. With a temperature probe, students can
investigate the relative temperature of parts of their bodies, the temperature of
hot water as it cools, or the cooling curve of hot water insulated by different
materials.
Microcomputer-based labs are a useful technology for elementary grades through
college. In the years that MBLs have been used in universities, evidence has
accumulated that students can gain a better understanding of difficult concepts
such as the derivative through using MBL. MBL-based curricula for young children
are now available as well.
3. Simulations
A related set of tool-like programs are simulations, software that allows a user
to change parameters and run a model to see the effect. Such software supports
students' building and testing of hypotheses, interpretation of results, and
subsequent revision and retesting. With Oregon Trail, for example, students play
the role of the head of a family heading West to settle. Numerous obstacles and
decision points arise on the way; different decisions about how much food to
take, how many miles to go in a day, and how to ford a stream lead to different
outcomes. The Search Series provides a variety of challenges - looking for oil,
searching for treasure on an island, etc. - that students explore in teams. Each
team decides on a navigational move, enters it into the computer, receives data
about what happened, and spends some time plotting its next move. The Search
Series is organized so that an entire classroom of students can use a single
computer; one team enters its new parameters while the others decide on their
next move and research the topics involved in the simulation.
Simulations differ from one another primarily in terms of the world that is
simulated and the complexity of the underlying model. Sometimes the simulated
world is the "real" one, as in predator/prey programs. In these, the educational
goal is for students to understand what relationships govern the real-world
behavior; that is, how the relative number of predators (e.g., foxes) and prey
(e.g., rabbits) affect one another. Students learn underlying principles such as
if the number of prey becomes very small, the number of predators will drop as
well because they have insufficient food. They also learn what the mathematical
forms look like that describe such behavior (e.g., exponential, and logarithmic
curves).
Sometimes a simulation actually expands the world available to a student, because
the world that is modeled is one that is otherwise inaccessible. RelLab (Horwitz
& Barowy, 1994) is such an example. In this case, the educational goal is for
students to learn about relativity, and the effects of motion at and beyond the
speed of light. Obviously, no student can experience this phenomenon or
experiment with it other than through a computer simulation; therefore, the
technology serves a particularly valuable role.
Other simulations let students investigate an entirely imaginary world that
behaves according to well-founded principles derived from real-world behavior.
The very popular Sim series (SimCity, SimTown) is such an example. In each of
these, students build a city (or town, etc.) of their own design, then "run" it
as mayor, making decisions about building, spending money, raising taxes, etc.
These are popular pieces of software, played frequently as games in students'
homes. But the reasoning and planning that they encourage fits easily under the
rubric of "inquiry." .
4. Multimedia
All the categories described above can be enhanced by the addition of multimedia;
that is, computer-based video or audio. As these capabilities become more
available and more affordable, the buying public will expect more sophisticated
pictures and sounds to be a part of every piece of software. Of course,
multimedia is not educational in and of itself. But, used well, it can help bring
the world into the classroom in ways that are authentic, motivational, and deeply
inquiry oriented. Unfortunately, many "educational" uses of multimedia just add
"art" to text in the same way textbooks add illustrations to make the page look
more interesting.
Multimedia makes the most of its educational potential when students actually use
the medium as data (e.g., CamMotion), when they have to create an argument based
on synthesizing different pieces of video (e.g., comparing Orson Welles and John
Huston as directors, illustrated with clips of several of their movies), or when
the video provides a context for a particularly interesting problem (e.g., Voyage
of the Mimi, Jasper). Some particular combinations worth noting are
data-collection tools that operate on multimedia (CamMotion), curricula that use
video as an anchoring context (Jasper), and content-based multimedia "tours" that
work in both school and informal settings such as museums (Palenque).
CamMotion is software developed as part of the VIEW (Video for Exploring the
World) at TERC (Rubin, 1993). It allows students to collect data such as
position, distance, and angle that changes over time. It allows a student to
explore, for example, the flight of a ball thrown from one person to another or
the position of a girl's legs as she does multiple cartwheels; the student clicks
on the relevant spots on the screen, then composes a graph that corresponds to
the motion. The student can then point to various parts of the graph and see the
video that corresponds to that mathematical representation.
CamMotion can also be used with videos that students or teachers make. Taking
videos can be combined with mathematical representations, for example, by having
teams of students take videos that illustrate a particular graph. A graph of
smooth deceleration, for example, might evoke videos of students sliding in their
socks, a ball going up a ramp, a ball thrown into the air, a toy car at the end
of its rubber band, a car stopping at a traffic light, a pendulum at the top of
its swing. This software greatly extends the possibilities for student data
collection and representation by making the visual and kinetic world of the
student available as sources of data.
Jasper (Cognition and Technology Group at Vanderbilt, 1990) uses video to present
a narrative situation that poses challenges or questions that the principal
character needs to solve. Designed for middle school students, Jasper includes in
the narration all the relevant information needed to solve the problem. The
classroom interaction is heavily prescribed by the program to support active
learning on the part of the students, supported by guided inquiry techniques on
the part of the teacher. Here the video is a partner in the inquiry experience
with the teacher. Much of the burden of setting up and shaping the
problem-solving situation is on the teacher, but the video provides the
opportunity for students to connect with the video, see salient problems in the
characters' actions, and work on those problems with the information presented in
the video. The video itself provides an experience that provides a common context
for discussions between and among students and the teacher.
Palenque (Wilson & Tally, 1991) is a videodisc and accompanying software that
allow students to explore a Mayan ruin in southern Mexico in an experience
sometimes called "virtual travel." Slides, film, video, graphics, text, sound
effects, and audio narration are all integrated on a single videodisc, designed
to be totally student directed, rather than dependent on a teacher's
instructional sequence and objectives. Students have simulated travel tools, such
as a camera, a photo album, and a compass that allow them to navigate and collect
snapshots of where they have been. An 8-year-old Mayan "specialist" provides
informed commentary on the videotape.
Palenque was designed primarily as a prototype and has not been used much in
school settings; therefore, we know little how it would interact with a school
curriculum. Primarily, it provides us with an indication of how technology might
offer information in a way that is compelling to individual users and fosters
sense-making activities, rather than just browsing. Note that achieving this goal
was difficult and required a multiyear development effort. In the end, it is a
breath-taking experience to use Palenque, and its potential to be further
developed and used escapes no one.
There has also been a huge explosion of educational CD-ROMs on the market that
provide a set of images and short video clips on a single topic. Some examples
(these are from Tom Snyder Productions, but there are many other similar
offerings from other companies) are Rainforest Researchers, The Great Ocean
Rescue, and The Great Solar System Rescue. The experience of using these is
similar to the Jasper system described above; however, the curriculum and teacher
support are more limited because these are packaged commercial products - the
Jasper series is an ongoing research project that has continued to develop
supporting materials.
Each of these is a simulation that involves students working in teams to get
clues from the computer, deciding as a group on their next move, and then working
again as a team to analyze the results of their decision and to plan their next
action. The simulations are supported and extended by still clips, videos, and
print materials.
5. Educational Games
Games are a large category of software, as can be seen from a quick look through
any software outlet. Most games fall into one of two categories: action games
that rely mostly on hand-eye coordination, and drill games that resemble
automated flashcards with fancy scoring mechanisms and rewards. Some of the most
well-known action games are the good old Pacman (and all its variations), Tetris
(which has an added component of geometrical skill involved), and Brick-Out
(which requires some strategy as well as straight shooting). One of the most
common drill games is Math Blaster, in which the user has to shoot down facts
like 3 + 4 by quickly typing an answer. Some of these drill games are popular in
schools, where teachers use them for drill and as a "reward" for students,
suggesting their high motivational power.
One may sometimes have difficulty deciding when a piece of software is a
simulation or tool and when it is a game. Many of the most interesting games -
and in particular, those that show up most frequently in schools - are
simulations. Some of the most well known of these, which are described in detail
above as simulations, are Oregon Trail and SimCity. As mentioned above, the main
differences between these game programs and ones that are "officially" considered
simulations are the amount of connection to curriculum supplied with the software
and the role of a scoring system and "winning." For example, the Search Series is
different from Oregon Trail mainly in that the former is built around educational
content specifically designed to connect with curricula, and the latter trades
off curricular content for entertainment value.
Other kinds of games also have significant educational content. The popular Where
in the World Is Carmen San Diego? (a game in which players try to find Carmen by
following geographical clues around the world), for example, supports students'
learning about geography. The Most Incredible Machine invites students to create
Rube-Goldberg machines that can get the ball in the basket, start the motor, for
instance, by combining a wide variety of objects, gears, pulleys, etc.; this game
supports students' general problem-solving and elementary physics abilities. A
new game, The Incredible Logical Journey of the Zoombinis, creates a fantasy
world in which students need to use reasoning in discrete mathematics to get a
band of characters to Zoombini Town safely. The very popular Myst uses elegant
video and sound to enhance a challenging adventure-type game that requires
problem solving and arcane thinking.
One might safely say that much more work has to be done in the arena of games. A
predominance of violence in the subject matter and action of most games still
exists, which makes them more appealing to boys than girls. Many game designers
spend more time on flashy rewards than on educational content. There are few
games that require knowledge of algebra, fractions and decimals, or biology, for
example, pinpointing a need for substantial development in the future.
6. Cognitive Tools
Cognitive tools are a varied group of software that take advantage of the power
of the computer to present "concrete" representations of abstract concepts. The
main components of cognitive tools are an underlying numerical model that can be
changed by the user and a visualization of the behavior of this model that is
determined by "running" the model with the user's choices of values. In some
cases, the user's goal is to match a particular behavior of the model by changing
numerical settings in the model. In others, the user must match a particular goal
state by manipulating the representation itself. The Shifty Lines software
described above is an example of the second kind of tool; students explore the
best fit regression line by moving a line around on a scatterplot. The
coefficients of the line and a measure of the sum of squares are represented
numerically on the screen and change as the line is moved. The students' goal is
to find the line for which the sum of squares of the residuals is smallest then
to explore how deleting individual points affects the least-squares line.
An example of a tool with which students try to match a goal action by changing
variables is the Envisioning Machine, a program designed to portray a graphical,
dynamic simulation of an expert mental model of velocity and acceleration. With
this software, the user manipulates a Newtonian World shown on one half of the
screen in which an object is represented with velocity and acceleration vectors.
The other half of the screen is taken up with an Observable World which shows a
motion as normally viewed. The goal of the user is to match an Observable World
motion by setting the appropriate velocity and acceleration vectors in the
Newtonian world.

Teasley and Roschelle (1993) analyze a long episode of collaboration between two
15-year- old boys trying to create the motion of a ball being thrown up into the
air, slowing down, and then falling by the force of gravity. It was the first
problem they had encountered in which the velocity and acceleration vectors
pointed in opposite directions. Early in the collaboration, the two boys agreed
that the velocity vector had to point up, and the acceleration vector point down.
They disagreed, however, on how to figure out the lengths of the two vectors.
Through their discussion of how to set the initial speed so that the ball reached
the appropriate height, both boys significantly improved their understanding of
the interaction of velocity and acceleration. A reading of the transcript
demonstrates that neither could have completed the task as successfully alone.
This incident shows that, although software can be a critical catalyst for
learning, inquiry relies on more than just software. If each of the boys had
worked alone on the same problem, they both would have learned much less. The
conversation between them and their teacher's guidance in choosing the problem
and making carefully chosen comments as they worked allowed the potential of the
software to be realized. Software designers who understand these dynamics design
their tools to support collaboration by including such elements as runnable
models and visible goals.
Many cognitive tools also make use of linked representations, such as the line
and the coefficients in Shifty Lines. We would normally expect the line to change
when the user defined new coefficients. In this case, the opposite is true: the
coefficients change when the line moves. This shift allows students to experience
the concept of least-squares fit from a visual perspective and to carry out
"what-if" experiments by direct manipulation of a graph.
These ideas are part of a software design movement that gives growing prevalence
and importance to multiple linked representations. The growth of this kind of
representation is due to both theories of multiple intelligences put forth by
Howard Gardner (1983) and observations of students learning with such tools. The
idea is that many concepts, particularly in mathematics, have representations in
different modalities, pictorial, symbolic, numerical, graphical. The
relationships among these representations are an important kernel of the
knowledge students need to gain about the underlying concepts, as in mathematics,
for example. In mathematics programs that use multiple linked representations, a
parabola might be represented in an animation, an equation that specifies slope
and intercept, a table of points, and a graph; but, if the student changes one of
these, all the others will change in response. This introduces new task
possibilities, such as "Change the slope and intercept of a line to get a certain
table of points" or "Change the animation vectors to get a certain graph of a
character's speed." Even though multiple linked representations are especially
common in cognitive tools, they are becoming increasingly common in tools and
games, because they provide effective support for inquiry in two ways. They
provide the multiple paths to understanding that we know work for different
students and make accessible the relationship between representations that foster
the understanding of many scientific domains.
7. Intelligent Tutors: Sources of Scaffolding
Scaffolding is an educational term that reflects the physical object for which it
is named. Scaffolding is a temporary support system provided by a teacher to help
students accomplish a complex task. As the student becomes more accomplished, the
scaffolding "fades," just as scaffolding on a building is taken down once the
construction is complete. Scaffolding can be as simple as a teacher helping
students do complex computational problems in steps and keeping track of the
results for them. A more complex scaffolding might involve setting out a specific
strategy for completing a research task. In both cases, the student will
eventually have to do the task alone, and it is the teacher's job to withdraw his
or her support as the student is able to work independently or with other
students.
For years, artificial intelligence researchers who have tried to make computers
"smarter" have dreamed of developing intelligent tutors that could scaffold
students' learning, providing help just when it was needed. To accomplish this,
such a system would understand students' answers, figure out what they understand
and where they are having trouble, and decide what problem to pose next and what
advice to offer, all without a scowl or recrimination: in short, serve as the
"perfect" teacher. One argument that has been made in favor of such intelligent
tutors is that this use of technology would give students individual attention in
subjects in which there may not be enough teachers to go around.
Ideally, an intelligent tutor includes the knowledge that experts have in a
domain (e.g., geometry), the ability to use and explain this knowledge, the
tutoring ability that guides when and how to interact with the student, and some
ability to understand what the student does and does not understand at a
particular moment. These systems are often based on research that seeks to find
the relationship between the rules that novices use and those that experts use.
Designers then develop the software to help students acquire the rules they might
be missing - and the strategy to use them.
The first program in the intelligent tutor style was Buggy (Brown & Burton,
1978), which could find patterns in errors students made in subtraction and was
seen primarily as a way for teachers to learn how to do this kind of pattern
search on their own. Since then, several topics have been popular subjects for
intelligent tutor production: math (especially geometry and algebra), computer
programming, and adult industrial training such as electronics testing (Lesgold
et al., 1989). An example of such software is that of Anderson, who has written
several tutors for high school geometry proofs. The figure below illustrates the
way these tutors may be set up (Lajoie & Derry, 1993).
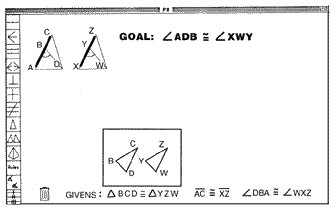
In this case, the given information that two triangles are congruent, that two
line segments are congruent, and that two angles are congruent are all given on
the bottom of the screen. The goal and a diagram of the givens are shown at the
top. Suppose the student does not know what to do at this point in the proof. The
intelligent tutor can give a hint, based on its understanding of the structure of
the proof. In this case, the tutor might "realize" that the student needs to
prove that triangles ACD and XWZ (the large ones) are congruent in order to show
that angles ADC and SWZ are congruent and, as a result, that angles ADB and XWY
are congruent. The first hint this tutor would offer is "Find proven part
statements of triangles ACD and XWZ and use them to justify the congruence." If
the student still had trouble, he or she could get the next hint: "Justify the
congruence of triangles ACD and XWZ using the statements AC is congruent to XZ,
DC is congruent to WZ, and angle DCA is congruent to angle WZX." If a student is
unable to follow even the most directive hint, he or she may be told to ask the
teacher for help (Koedinger & Anderson, 1989, 1990).
The scaffolding that such programs offer aims to provide students with more
opportunity for inquiry by helping them to move ahead over potential sticking
points and by managing some of the attention-draining details of complex
problems. In some content areas, this strategy can work; in particular,
well-defined, well-structured areas of knowledge, in which not only the knowledge
but how to apply it, can be expressed in terms of rules. The geometry proof
example above is well-suited, because both the geometry proof rules and
strategies for applying them are easily coded in deterministic sequences. A
danger, though, is that the program manages just those aspects of the task that
are the heart of inquiry: deciding on the next step to pursue, ordering steps,
exploring alternate inquiry paths. After all, doing proofs does at some level
require planning the approach. In addition, more loosely defined subject areas,
such as constructing appropriate representations for data, researching and
describing the plight of Native Americans in the 1600s, or writing a
communicative, persuasive letter, do not fit as well into an intelligent tutor
structure.
Thus, the vision of intelligent tutors appears to be only partly fulfilled. As
these systems have become more sophisticated, the gap between their knowledge of
the subject matter (which can be quite good) and their effectiveness as teachers
(which typically leaves much to be desired) has become increasingly apparent
(Lepper et al., 1990). The original intent of providing computer-based
scaffolding has merit, but it may have more potential when viewed as adjuncts to
tools (as in the Tabletop example given earlier) that facilitate discussion among
students and teachers.
8. Construction Environments
"Constructionism" is an educational philosophy that is an extension of
constructivism; constructionism adds to the picture of learning an emphasis on
students creating just about anything - stories, physical structures, computer
games, drawings - with appropriate tools that require significant thought. As a
theory of learning, it based on the belief that students learn best when they are
designing and constructing objects (including computer procedures) of their own
imagination and desire. The most famous example of environments in which students
learn through creating their own objects is LOGO, a programming language created
in the 1960s and championed by Seymour Papert (1993). LOGO and the companion
Lego/LOGO (in which Lego toys are controlled through a special cable connected to
a computer running LOGO) can be catalysts for experiences in which students learn
mathematics, simple machines, gear principles, etc. However, when it was first
introduced, LOGO tended to be used in situations with little guidance from a
teacher. Unfortunately, this misunderstanding about how LOGO should be used led
to experiences in which its educational potential was seldom realized.
Today, LOGO tends to be used in more directed settings, so that students'
exploratory behavior is more likely to be guided. One LOGO application that
contains considerable curriculum support can be found in the Investigations math
curriculum, where a special version of LOGO called Geo-LOGO forms the basis for
two-dimensional geometry units in Grades 3 through 5. These units approach
traditional content in a nontraditional way. In third and fourth grades, students
learn about rectangles through using LOGO to draw rectangles of different shapes,
sizes, and orientations. Rather than memorizing a definition of the word
"rectangle," they discover properties of rectangles by programming the computer
to draw them.
The fact that opposite sides of a rectangle are the same length comes to life
when one has had to figure out that if one side is drawn by FORWARD 30 (a command
that draws a line 30 units long), the opposite side must have the same command
(or the equivalent, e.g., FORWARD 15, FORWARD 15).
Another variation quickly gaining in popularity are MUDs (Multi-User Domains) and
MOOs (Multi-User Domains with Object Orientation). These odd names refer to
gaming environments that are built collaboratively over networks. A game grows by
players adding interconnecting rooms constructed with tools that come with the
game. Each player can also construct his or her own identity for a particular
game, choosing an age, physical characteristics and possessions. To play, a
participant visits rooms, asks questions about objects, picks up objects (if
allowed by their creator), and possibly solves riddles. Sometimes passage to
another room depends on the player having a certain collection of objects. To
build a room, a player must use a kind of programming language to specify the
appearance and behavior of objects in the room. These environments are more often
than not used outside school, but the kind of building that they require of
players is a sophisticated skill that could easily be harnessed in school.
MUDs and MOOs are not only construction environments; they are also communication
environments. Much of the thrill for the player lies in watching other people
maneuver through his or her room and trying to solve other people's riddles. The
kind of communication that happens over networks in these games is one example of
a much more widespread class of software that support connections between people
over distance.
9. Virtual Communities
Ever since the Internet became available to the general public just a few years
ago, its connections have hummed with personal, business, and educational
messages. The World Wide Web, which carries pictures, sound, and videos along
with text, has expanded the kinds of communication that can occur. There are many
educational innovations that take advantage of these developments. I discuss them
here in two groups: innovations that are primarily about communicating text and
those that focus on sharing data. Of course, there are examples that involve both
text and data as well as examples such as MUDs, that center on sharing
constructions. Because about anything (that isn't too big) can be shared over the
World Wide Web or the Internet, there could be many more categories, but these
two foci are useful because they support a useful grouping and comparison of
software.
Sharing Text: Communication Environments
Computers have been providing and supporting enriched communication environments
for a considerable time. In the early 1980s, when word processors on
microcomputers were beginning to appear in schools, the ease with which students
could edit and publish their work made their writing more authentic. Educators
realized that by using word processors their students could write with real
purpose to a real audience, making the experience more genuine and engaging both
writer and reader in a richer process. Current educators sometimes talk about
"electronic communities" in which a common interest or project or both unites a
set of geographically dispersed participants in a generative communicative
structure.
The QUILL software (Bruce & Rubin, 1993) was a set of writing tools and
environments developed in 1982-84. It included a library environment where
students could share their writing with others, a mail environment which was a
primitive e-mail system, and a planning tool in which students could construct
and use planners that helped them organize their writing. The software was used
in the context of curriculum influenced by the Bay Area Writing Project, which
focused on the writing process and writing conferences. In line with the writing
process emphasis, many QUILL classrooms published class newspapers, literary
journals, or poetry collections. One school in a small village in Alaska even
published a calendar that included the birthdays of every resident in the town,
decorative drawings by the school children, and stories in both English and
Athabascan. A not-surprising result of the QUILL field test: QUILL's success in
fostering improvement in students' writing was related most closely to how well
the suggested curriculum was implemented. Teachers who stuck to a more
traditional approach to writing that emphasized grammar and allowed little
opportunity for revision saw fewer gains in their students' writing.
Today, the opportunity for technologically enhanced communication environments is
orders of magnitude greater than it was 10 years ago. The Internet and the World
Wide Web provide potential audiences all over the world, across cultures and
continents. But it has taken a while to get the social structure right. In the
early days of using the Internet in education, teachers often set up pen pals for
their classes in other states or countries. However, people soon discovered that
the pen pal mode did not always work out well. There were never the right number
of students in the two classrooms for a student-to-student match, and after the
initial exchange of information, students often had nothing to talk about. Since
then, other communication models have been developed. A common one is a centrally
organized project uniting a small cluster of classrooms into an activity that
gives focus and purpose to their communication. For example, AT&T Learning
Circles (Riel, 1995) pull together a group of classrooms into a several months'
long project, such as producing a collaborative newspaper. An editorial team for
each page is composed of students from all four classrooms; they use the network
to submit and discuss articles, respond to submissions by other authors, and lay
out pages. The final product is interesting to all participating classrooms
because it contains articles on local activities they recognize and articles
about the other schools and students they have been getting to know. Notice how
the success of such an activity depends as much on the social organization of the
writing process within and across classrooms as it does on the software per se.
It is also interesting to note that these kinds of computer uses - in which
communication and writing are at the center - are some of the most popular with
girls. The use of computers to start and develop interpersonal relationships is
one that girls find particularly appealing (as do many boys).
Another social structure that works well is a "virtual forum," in which
interested students share information through electronic mail or a bulletin board
on a topic of mutual interest. If these bulletin boards are organized through a
project, there may be experts - scientists, mathematicians, writers, artists, for
example - involved, so that students can discuss their questions and share their
work with adults who work in the profession. Such a connection with real-world
audiences not only adds legitimacy to students' work, but also provides them with
role models of people who use the skills students are developing in their daily
work.
An active area of work right now is the attempt to include parents in these
networked- based communications environments. Some of the more pedestrian uses of
such an environment would be to keep parents informed of their students' homework
assignments and attendance. But more interesting applications might be to involve
parents in the same kinds of inquiry students are pursuing; that is, contributing
to a history of the town or to a discussion of water quality issues. The more
people in a community (network based or not) who are practiced at inquiry, the
more it will be valued and supported.
Networking as Data Communication
Many educators have seen networks as important tools in providing more meaning
for data collection. If values for data differ according to geography, then
disseminated data collection and sharing allow students to discover interesting
contrasts that they would not otherwise be able to see. National Geographic Kids
Network® is one of the first and best-known examples of using networks to
communicate data; it links students all over the United States and in a number of
foreign countries to work collaboratively on science projects. In each project,
students collect data and send them to a central source where they are combined
with other data; the larger data set is then sent back to each school. The first
two units of the Kids Network curriculum are Hello! (an introductory unit in
which students share information about their pets) and Acid Rain (a science unit
in which students collect, collate, and compare data about the acid content of
water sources in their community). Other units examine weather, trash, solar
energy, and nutrition. An evaluation of this curriculum-technology combination
showed that students gained in their ability to organize, represent, and
interpret data. They also gained in specific content areas related to their
associating data with different locations, e.g., the ability to use latitude and
longitude.
Global Lab is a project in which schools are connected worldwide to study and
compare environmental data. This network also includes several earth scientists
who consult on projects and on the interpretation of data. The GLOBE project
(Global Learning and Observations to Benefit the Environment) is a similar
endeavor that hopes to link students in more than 2,000 schools around the world
in studying the earth's environment. Projects such as these are often called
"telecollaborations" because they allow people to use telecommunications to
collaborate across space and time. With appropriate curriculum and teacher
support, these projects can support and extend inquiry by providing data, tools,
experts, and audiences for student's scientific exploration.
10. Information Access Environments
The Internet, the World Wide Web, and a quickly growing number of reference
CD-ROMs (e.g., Encarta and Compton's Encyclopedia) have provided students with
seemingly infinite access to information and expertise. Some of the most zealous
technophiles make the argument that because information is growing exponentially
and teachers cannot know everything, connecting students to the primary sources
of information is necessary for their education. Access to the resources of the
Internet and the World Wide Web is one of the major arguments given for adding
technology to schools.
Unfortunately, giving students unstructured access to the Internet is similar to
letting them loose in the library - a lot more pedagogical structure is necessary
for learning to happen. In the worst case, students can copy from the network
into an online homework report; here the technology has simply made that process
much easier than copying from a traditional encyclopedia ever was. Yet, we have
all seen students who are excited by the ease of access in online environments,
and by the video and audio that accompany many entries. The challenge is to turn
this motivation into real learning to avoid burn-out through lack of content.
The educational structures that make the best use of these new resources are
project-based learning, in which students are engaged in collaborative research
they have helped to define. In these cases, students can use the Internet's
information tools in the context of a larger goal, so that deciding which
resources to use, how to find them, and what information to extract are all
related to a project. In the context of such projects, the Internet also provides
electronic communities in which scientists, historians, mathematicians, and
others are willing to answer students' questions through programs such as Ask A
Scientist and Ask a Mathematician to which schools can subscribe.
One problem that arises when students use network connections for research is
that a text document is seldom the most appropriate structure for them to express
their results. If they find drawings, pictures, or videos to support their
statements, they will naturally want to include them. Several multimedia document
programs for use in schools have appeared in the last few years (e.g., Mediatext
and Hyperstudio), and they are bound to become more widespread as access to the
Internet's multimedia resources continues to grow. Besides supporting the
construction of multimedia documents, some of these tools allow students to put
together hypertext documents in which each reader can read different parts of the
document, according to interest, by following particular links. These uses are
closely connected to the next category, information construction.
11. Information Construction
A focus on information construction comes from seeing students as constructors of
their own knowledge, rather than as consumers of other people's knowledge. This
category embraces a range of applications, from the use of a computer to make
interactive multimedia reports to having students in a classroom create their own
"encyclopedia": a classroom knowledge base recorded on the computer. The most
well-known example of the latter approach is the Computer-Supported Intentional
Learning Environments (CSILE) system (Scardamalia, 1989). In a CSILE classroom,
one of students' main activities is using software that prompts them for ideas,
facts, implications, for example, then for connections to other students'
contributions. Embedded in the software are tools that help students structure
and record their questions, approach, and results. In particular, students are
asked to label the pieces of information (called "nodes") they enter as
"problem," "my theory," or "new information." These labels help them notice,
understand, and use the structure of an argument in building their collaborative
database. This software differs from existing data sources (e.g., the Web) in
that students create their own materials. Consequently, there is no temptation on
students' parts to merely copy what they have read.
CSILE is a prime example of software whose use must be supported by the
curriculum and the teacher. At first, students do not understand how to build
their own knowledge base, nor do they distinguish between the various labels. The
first weeks and months of using CSILE must be carefully scaffolded by the teacher
so that the classroom database and the students' knowledge grow together.
12. Computer-Assisted Instruction and Integrated Learning Systems
Computer-assisted instruction and integrated learning systems were some of the
earliest applications of computers to education. CAI's roots are in behaviorist
theory and programmed instruction; it delivers drill-and-practice exercises on
basic skills and immediate feedback on student performance, combined with either
a teacher- or computer-delivered lesson on the topic. Because computers can hold
large numbers of problems and can execute the simple logic for deciding on the
next question, some CAI programs individualize instruction for each student. From
a CAI perspective, students are seen as consumers of "facts" that can be
delivered by machine as well as by teachers.
Because the content and format of CAI (with multiple choice, true/false, or
fill-in-the- blank questions) is so similar to that found in standardized tests,
studies of CAI often show student gains, especially if students have had frequent
exposure to focused CAI over at least a semester to a year. However, there is
less information about the effect of these CAI experiences on the development of
other skills and understandings that new assessments are targeting. Because CAI
is not designed to support inquiry activities, it would be inappropriate to
measure its effect with performance-based assessment tools.
There are some additional concerns about CAI software. This kind of program is
referred to as "drill and practice," but it is often colloquially called "drill
and kill." Why? Because repeating a set of problems by rote may contribute little
to students' intellectual development; in fact, it has the potential to kill
their interest in the topic in question. Their engagement is often based on
special effects rather than intellectual involvement. Their activity does not
contribute to their ability to analyze more complex problems. For example, middle
school students who had long before mastered simple addition and multiplication
facts have been observed in their free time playing Math Blaster, answering
questions far below their mathematical level, just for the sense of simple
success and for the special effects.
Note that some CAI programs are marketed to homes as well as schools. Typing
Tutor and Math Blaster are two that are frequently seen in students' homes.
Catalogues are full of Math Blaster look-alikes that drill students on basic
arithmetic. Parents even buy these for their children, thinking the software will
help them in school. It is often hard to dissuade parents from doing this,
because the software looks exactly like the tests parents remember from school -
they are not aware of the changes in education encouraged by such movements as
the NCTM standards for mathematics.
Integrated learning systems include both courseware and management software and
run on networked hardware. They cover one or more curriculum areas across grade
ranges, and usually include a management program that tracks and reports student
progress. The more traditional integrated learning systems are in fact
collections of drill and practice sequences in various areas and skill levels,
similar to the CAI programs discussed above. Some of them are adaptive; that is,
they present students with problems of differing difficulty depending on how well
they have done on previous problems (Van Dusen & Worthen, 1995).
More recently, integrated learning systems have begun to add materials that
require students to solve more complex problems that foster deep reflection and
genuine understanding. Such integrated learning systems typically include
software such as science simulations, as well as tools such as word processing
programs, spreadsheets, graphing packages, and encyclopedias. These integrated
learning systems are moving away from the traditional definition of computers as
machines that offer the opportunity for programmed instruction and management and
toward seeing the computer as a set of tools. Two major differences, however,
between an ILS and a set of tools are that an ILS includes a highly structured
and relatively inflexible curriculum and that it usually runs on dedicated
hardware and thus may preclude any additional uses of the computers.
Although integrated learning systems are designed to have students work
independently, teachers need to integrate ILS work with classroom activities for
these systems to have a maximum effect on student learning. Integration with
classroom activities can require considerable creativity in the ILS scenario.
Even though the ILS is capable of "individualized" instruction, letting students
function at different grade levels at the same time, teachers still have to deal
with their students being at different levels in noncomputer class time. In fact,
because students have been working on totally different problems during ILS time,
whole class activities can be even more of a challenge than they might be
otherwise. Teachers have to make use of the same kinds of techniques that are
necessary in a classroom without computers - small group work and teachers
working as facilitators and coaches rather than lecturers. Therefore, even in a
computer-intensive, highly structured environment, we see one more example of
theme: student learning depends on teachers and curriculum at least as much as on
the hardware and software.
The following succinct conclusion about CAI and ILS comes from Jones et al.
(1995, under review):
Closed electronic models such as the typical CAI and ILS that support
traditional learner goals, curriculum, instruction, and assessment are
fundamentally not much improved over the traditional models. New research on
learning is saying that traditional models of learning and schooling, like
dinosaurs, are not adaptive to the needs of modern society. To the extent these
technologies support traditional teaching and learning, they are misaligned with
educational reform and the needs of the 21st century. (p. 10)
A Final Note: Computers in Multicultural Education
Unfortunately, all these resources are not equally distributed. Equity is a
problem everywhere we look in education as it relates to technology: students of
lower-income families have less access to technology in school and often use it
in more constrained ways; girls lag behind boys in math, science, and technology;
students of color in primarily inner city schools are less likely to have access
to computers outside school. In fact, access to information may become the new
definition of educational equity (Office of Technology Assessment, 1995b).
Computers are a double-edged sword in equity matters: they can be a new way for
some students to have a voice and find expression for their talents, or they can
add to the growing distance between the haves and have-nots.
In school, poor and minority students tend to use computers in less independent
ways than upper middle-class white students, with ILS and CAI uses being more
common than the tool- based approaches more affluent students enjoy. Being female
is a disadvantage in either case; girls do not use computers as much either in or
outside school, and their parents, when they do buy computers, often buy
different kinds of software for their daughters than they do for their sons
(Carol Edwards, personal communication, December 1995).
But there are several hopeful stories. The National Foundation for the
Improvement of Education (1995) provided fellowships to several teachers who were
interested in using computers in the service of multicultural education in
classrooms that were inner city, primarily nonwhite students. Here are adapted
excerpts from the story of one of those teachers:
Hazel Lockett teaches English at an urban junior high in East Orange,
New Jersey. Her junior high has been designated an arts and music school. Most
students are black, but more than a quarter of them were foreign born, from the
Caribbean, Central America, South America, and Africa.
Ms. Lockett is convinced that the cultural differences among her
students significantly affect how they learn. In particular, she noted that some
of her students "want to blend in with the background when called on." She
worked in her class to find ways to bring out these quiet students. Her main
tools were expressive writing and the power of literature.
For her project, Ms. Lockett used Hyperstudio. She designed a project
for a group of 20 students based on Kindred, a science fiction novel by Octavia
Butler that tells the story of an interracial couple time-traveling to the South
of 1850. Using Hyperstudio, students created a multimedia stack to illustrate
the novel's themes around gender, race, power, and authority and to offer a
resource for multicultural education for future seventh and eighth graders.
Ms. Lockett observed a marked change in the patterns of interaction
among her students, both in and outside class. Racist jokes were less common and
cooperation grew. Formerly quiet students found their voice and some grew
significantly in self-confidence. She noted that the increased technological
competence students felt helped them believe in their ability to
learn.
She felt that the technology itself was helpful to several students
who were not outspoken or came from cultures with different attitudes about oral
speech. Oral class participation is a conventional avenue to success in American
schools, but it is especially difficult for some students. Technology makes it
possible to create a new classroom culture and new avenues of communication that
are unlike either oral discussion or traditional kinds of written
work.
The NFIE program is just a beginning, but it does point to some ways
technology may be able to enhance multicultural education and give students who
have less rich access to computers a chance to feel that computers are tools that
they, too, can use in powerful ways.
IMPLICATIONS FOR STUDENT ASSESSMENT
The use of computers in student assessment is a large topic in and of itself. As
performance assessment - which evaluates students based on the actual work they
are doing in schools, rather than a specially designed test - becomes mode widely
adopted, technology will become more important in supporting it. Sheingold and
Frederiksen (1994) note five functions of technology that support new kinds of
assessment and link them with educational reform:
- Supporting students' work in extended, authentic learning activities
- Creating portable, accessible copies of performances and replaying performances in multiple media
- Providing libraries of examples and interpretive tools
- Expanding the community of assessment participants
- Publishing selected student work and thus recognizing their accomplishments.
More detail on these and other connections between technology and assessment can
be found in the work of Sheingold and Frederiksen (1994).
IMPLICATIONS FOR TEACHERS: TOOLS AND PROFESSIONAL DEVELOPMENT
Tools
Teachers are more likely to use computers effectively in their classrooms if they
feel they are useful tools for their own work. Certainly, there are many ways in
which teachers can use basic tools such as work processors, spreadsheets, drawing
programs for their own purposes, both in and outside school. Spreadsheets are
useful for record-keeping; word processors for everything from writing letters to
parents to preparing materials for students. Using computer tools themselves
allows teachers to realize the power of computers to serve their own needs and to
become personally invested in their use.
Such straightforward uses can also grow into more dynamic classroom uses. Math
teachers, for example, may start by preparing text materials with a word
processor, but eventually they will want to write equations or draw graphs. They
may begin to use symbol processors or graphing programs, just for the output. But
it is impossible to use these kinds of tools without noticing how powerful they
are; soon some teachers will see the tools' potential and move toward using them
in more robust ways with their students.
The importance of allowing teachers access, time, and opportunity to make
computers their own tools is emphasized by Chris Dede (in O'Neil, 1995):
One of the mistakes that we made in implementing educational
technology was focusing first on students rather than teachers, because when the
computers on students' desks are mysterious devices to teachers, it's
unreasonable to expect effective integration into the curriculum.
Professional Development
The role of technology in professional development - whether or not technology is
part of the school change - is growing as more teachers have access to Internet
connections. Grant discusses this topic in detail; her paper deals with multiple
ways technology can support professional development for teachers, including via
distance learning, using videos to expand the realm of observation, creating
electronic communities of learners, and supporting teachers' daily activities
(see Professional Development in a Technological Age).
CONCLUSION
This paper suggests that there are many ways that technology can support
inquiry-based learning. The richness, flexibility, and power of computers and
their attendant technological kin offer valuable tools to support this approach
to teaching and learning. However, as the paper makes clear, the most powerful
variables are the teacher and the overall curricular goals of the school.
Technology is only as powerful as the way it is used. The choice is up to the
educators.
REFERENCES
Berenfeld, B. (1996, April). Linking students to the infosphere. Technological
Horizons in Education Journal, 23(9), 76-83.
Brown, J. S., & Burton, R. R. (1978). Diagnostic models for procedural bugs in
basic mathematical skills. Cognitive Science, 2, 155-192.
Bruce, B. C., & Rubin, A. (1993). Electronic quills: A situated evaluation of
using computers for writing in classrooms. Hillsdale, NJ: Lawrence Erlbaum
Associates.
Edwards, C., National Foundation for the Improvement of Education. (1995,
December). Personal communication.
Epie Institute. (1991). The latest and best of TESS, The educational software
selector, 1991 Edition. Hampton Bays, New York: Author.
Gardner, H. (1983). Frames of mind. New York: Basic Books.
Herman, J. L. (1994). Evaluating the effects of technology in school reform. In
B. Means (Ed.), Technology and education reform. San Francisco: Jossey-Bass.
Horwitz, P., & Barowy, W. (1994). Designing and using open-ended software to
promote conceptual change. Journal of Science Education and Technology, 3(3).
Jones, B. F. et al. (1995). Plugging in: Choosing and using educational
technology. Washington, DC: Council for Educational Development and Research,
North Central Regional Educational Laboratory.
Koedinger, K. R., & Anderson, J. R. (1989). Perceptual chunks in geometry problem
solving: A challenge to theories of skill acquisition. In Proceedings of the
Eleventh Annual Conference of the Cognitive Science Society. Hillsdale, NJ:
Lawrence Erlbaum Associates.
Koedinger, K. R., & Anderson, J. R. (1990). Abstract planning and perceptual
chunks: Elements of expertise in geometry. Cognitive Science, 14, 511-550.
Lajoie, S. P., & Derry, S. J. (1993). Computers as cognitive tools. Hillsdale,
NJ: Lawrence Erlbaum Associates.
Lepper, M. R. et al. (1990). Self-perception and social-perception processes in
tutoring: Subtle social control strategies of expert tutors. In J. M. Olson & M.
P. Zanna (Eds.), Self-interference processes: The Ontario symposium (pp.
217-237). Hillsdale, NJ: Lawrence Erlbaum Associates.
Lesgold, A. M. et al. (1989). An intelligent tutoring system for electronics
troubleshooting: DC-circuit understanding. In L. Resnick (Ed.), Knowing and
learning: Issues for the cognitive psychology of instruction. Hillsdale, NJ:
Lawrence Erlbaum Associates.
Michaels, S. (1990, Autumn). The computer as a dependent variable. In D. G. Lux
(Ed.), Theory into Practice, 29(4).
Mokros, J. et al. (1994). Assessment of investigations in number, data, and
space. [Unpublished manuscript]. Cambridge, MA: TERC.
National Foundation for the Improvement of Education (1995). Touching the future.
[Annual report].
Nickerson, R. S. (1988). Technology in education in 2020: Thinking about the
not-distant future. In R. S. Nickerson & P. P. Zodhiates (Eds.), Technology in
education: Looking toward 2020. Hillsdale, NJ: Lawrence Erlbaum Associates.
O'Neil, J. (1995). On technology and schools: A conversation with Chris Dede.
Educational Leadership, 53(2). Alexandria, VA: Association for Supervision and
Curriculum Development.
Office of Technology Assessment (U.S. Congress). (1995a). Future visions:
Education and technology. OTA-BP-169. Washington, DC: U.S. Government Printing
Office.
Office of Technology Assessment (U.S. Congress). (1995b). Teachers and
technology: Making the connection. OTA-EHR-616. Washington, DC: U.S. Government
Printing Office.
Papert, S. (1993). The children's machine: Rethinking school in the age of the
computer. New York: Basic Books.
Riel, M. (1995). The future of teaching. In Office of Technology Assessment (U.S.
Congress). (1995a). Future visions: Education and technology. OTA-BP-169.
Washington, DC: U.S. Government Printing Office.
Rosebery, A. S., & Rubin, A. (1989). Reasoning under uncertainty: Developing
statistical reasoning. Journal of Mathematical Behavior, 8(2), 205-219. Norwood,
NJ: Ablex Publishing.
Rubin, A. (1993, May). Video laboratories: Tools for scientific investigation.
Communications of the ACM, 36(5).
Scardamalia, M. et al. (1989). Computer-supported intentional learning
environments. Journal of Educational Computing Research, 5(1), 51-68.
Sheingold, K., & Frederiksen, J. (1994). Using technology to support innovative
assessment. In B. Means (Ed.), Technology and education reform. San Francisco:
Jossey-Bass.
Teasley, S. D., & Roschelle, J. (1993). Communication and collaboration: The role
of talk in children's peer collaborations. In S. P. Lajoie & S. J. Derry (Eds.),
Computers as cognitive tools. Hillsdale, NJ: Lawrence Erlbaum Associates.
Van Dusen, L. M., & Worthen, B. R. (1995, October). Can integrated instructional
technology transform the classroom? Educational Leadership, 53(2). Alexandria,
VA: Association for Supervision and Curriculum Development.
Walters, J., & Gardner, H. (1990). The development of education of intelligences.
In F. Link (Ed.), Yearbook on intellectual development. Washington, DC:
Curriculum Development Associates.
Wilson & Tally. (1991). Designing for discovery: Interactive multimedia learning
environments at Bank Street College. In B. Means & K. Olson (Eds.), Technology's
role in education reform: Findings from a national study of innovating schools.
Washington, DC: Office of Educational Research and Improvement, U.S. Department
of Education.
Additional Readings
Dewey, J. (1916). Democracy and education: An introduction to the philosophy of
education. New York: Macmillan.
Means, B., & Olson, K. (1995). Technology's role in education reform: Findings
from a national study of innovating schools. Washington, DC: Office of
Educational Research and Improvement, U.S. Department of Education.
Papert, S. (1980). Mindstorms: Children, computers, and powerful ideas. New York:
Basic Books.
Roschelle, J. (1986). The envisioning machine: Facilitating students'
reconceptualization of motion. In S. P. Lajoie & S. J. Derry (Eds.), Computers as
cognitive tools. Hillsdale, NJ: Lawrence Erlbaum Associates.
Rubin, A. (1991). Using computers in teaching statistical analysis: A
double-edged sword. In K. Sheingold, L. G. Roberts, & S. M. Malcom (Eds.),
Technology for teaching and learning. Washington, DC: American Association for
the Advancement of Science.
Ruopp, R., Gal, S., Drayton, B., & Pfister, M. (1993). LabNet: Toward a community
of practice. Hillsdale, NJ: Lawrence Erlbaum Associates.
Russell, S. J., Corwin, R., Mokros, J. R., & Kapisovsky, P. M. (1989). Beyond
drill and practice: Expanding the computer mainstream. Reston, VA: The Council
for Exceptional Children.
Van Dusen, L. M., & Worthen, B. R. (1992). Factors that facilitate or impede
implementation of integrated learning systems. Educational Technology, 32 (9),
16-21.
Weir, S. (1992, January). Electronic communities of learners: Fact or fiction.
Cambridge, MA: TERC.
|
 |

